74.09 0.23 5-32.32-65: A Comprehensive Guide to Understanding Complex Data Sequences

74.09 0.23 5-32.32-65 numbers are far more than just figures—they can serve as keys to unlock valuable insights. Take, for instance, a sequence like 74.09 0.23 5-32.32-65. While these numbers may seem arbitrary at first glance, they each carry significance that could shed light on underlying trends, patterns, and outcomes. Recognizing the meaning behind such sequences can greatly enhance how we interpret data, identify correlations, and make informed decisions across various domains.

Whether applied in scientific research, financial analysis, or broader data-driven fields, understanding the relevance of individual values allows for more nuanced insights and better predictive capabilities. This piece delves into how specific numbers might be interpreted and applied, emphasizing their importance in everyday decision-making processes.

Understanding the Meaning Behind Sequences of Numbers

In the world of data and analysis, numerical sequences can often seem perplexing, but they usually carry essential information that can reveal deeper insights. A string of numbers such as 74.09 0.23 5-32.32-65 may initially appear random, but each element of the sequence holds value. The combination of these numbers may serve a specific purpose depending on the context—whether it’s in data science, scientific studies, financial analysis, or even geographical systems. Understanding the purpose behind such numeric codes can significantly enhance our ability to interpret data accurately and make well-informed decisions in various fields.

Each component of the sequence contributes something unique to the whole, transforming it into a powerful tool for professionals and analysts across industries. These kinds of codes are essential for accurate measurement, reporting, and strategic decision-making, helping to reduce the complexity of data and making it more accessible.

How Numbers Tell a Story

In fields that deal with large datasets, numbers have an inherent ability to represent complex concepts in a condensed form. A sequence like 74.09 0.23 5-32.32-65 can hold diverse meanings based on how and where it is applied. At its core, this code is a structure—organizing numerical data in a way that’s easy to manage and interpret.

The first number, 74.09, might be a central data point or a reference measurement. This is often the starting point of the sequence and provides crucial context for understanding the rest of the figures. For instance, it could represent the temperature in a scientific experiment, a stock market figure, or even the latitude or longitude of a geographic location.

Next, 0.23 serves as a smaller, yet critical, value that complements or refines the primary data point. Smaller values like this often add precision, such as indicating a slight variation in measurements or providing finer details like a correction factor, a margin of error, or unit conversions. While seemingly minor, these values are just as important as the main number because they enhance the accuracy and reliability of the data.

Following that, 5-32.32 introduces the concept of a range, something that’s common in both scientific measurements and financial reporting. The dash between these two values suggests that the data can fluctuate within these limits. A range indicates flexibility, implying that the exact number is not fixed but falls somewhere between 5 and 32.32. This can be useful in cases where conditions are variable or when working with data that fluctuates over time.

Lastly, 65 provides the concluding element of the sequence. This final value might signify the completion of a measurement or the final point in a series of observations. It could be the end of a time range, a target value, or a critical figure that wraps up the entire dataset.

Taken together, these components form a cohesive unit that expresses much more than the sum of its parts. This structure is used across various industries to convey meaningful data that helps guide decision-making.

74.09 0.23 5-32.32-65

Numerical sequences like 74.09 0.23 5-32.32-65 are invaluable tools in data science, technology, and other analytical fields. They allow professionals to streamline complex information into digestible pieces. With the growth of digital technologies and the increasing reliance on data, such sequences are essential for managing and interpreting large datasets, whether it’s for predicting trends, calibrating measurements, or making real-time decisions.

These numbers are especially important in fields such as data science, where accuracy and precision are paramount. Whether it’s temperature readings, GPS coordinates, or financial data, using standardized formats like these makes it easier for analysts to detect patterns, track progress, and recognize anomalies in the data. For example, a sequence like this can simplify the task of processing vast amounts of information by breaking it down into manageable chunks.

The Role of Precision in Data Interpretation

In many cases, the numbers themselves are not the most important part of a sequence but rather how they relate to each other. The format and structure of the data—such as the presence of decimals and dashes—carry crucial contextual information. Take the numbers 74.09 and 0.23, for example. The first number is likely the primary measurement, while the second provides added accuracy. The two together give us a more complete and reliable interpretation of whatever is being measured.

It’s crucial to understand that in data analysis, even seemingly insignificant numbers can have a large impact on the overall interpretation. For instance, a slight difference like 0.23 could influence an experiment’s results or change how a business decision is made. In many cases, this attention to detail can help avoid major errors that could derail a project or lead to flawed conclusions.

Interpreting the Range: Flexibility in Data Sets

The use of a dash in the sequence, as seen in 5-32.32, is not just a formatting tool but a key indicator that the data is not static. The dash represents a range—a span of values within which the actual number may fall. This is crucial when dealing with dynamic environments or measurements that naturally fluctuate.

Ranges like these are particularly important in scientific research, where conditions often change over time or across different experimental setups. For example, temperature measurements can vary between a range of values, or a financial metric could fluctuate within a certain band. The use of ranges enables data to remain flexible and adaptable, which is essential for predictive modeling, risk analysis, and other applications that rely on varying conditions.

By acknowledging the possibility of fluctuation, the sequence acknowledges real-world complexity. In this way, it ensures that predictions or decisions based on the data are not rigid but can adjust as needed.

Why Accurate Data Is Key to Decision-Making

The importance of sequences like 74.09 0.23 5-32.32-65 extends beyond just providing clarity in data interpretation. They play a pivotal role in decision-making across different fields. Having access to precise, organized information helps decision-makers act quickly and confidently.

In fields like finance, even small data fluctuations can lead to significant changes in outcomes. Similarly, in scientific research, slight adjustments in measurements can affect the validity of conclusions drawn from experiments. A well-organized sequence of numbers helps reduce the risk of errors, ensuring that key decisions are based on the most reliable information available.

The compactness of these codes is one of their most significant benefits. Rather than sifting through long reports or complex datasets, decision-makers can quickly interpret numerical sequences, making them a crucial tool in fast-paced environments where timely decisions are vital.

From Complex Data to Clear Information

Understanding the components of a code like 74.09 0.23 5-32.32-65 requires not only breaking down the numbers but also recognizing their purpose in the larger context. Each part of the code has a specific function, whether it’s offering precision, defining a range, or providing a final value. Together, they allow professionals to manage and interpret data with greater clarity and confidence.

The ability to distill complex information into easy-to-understand formats is a skill that benefits individuals across various industries. Whether in the realms of scientific research, business analytics, or even daily technological processes, these numerical codes allow data to be communicated and understood with greater efficiency.

Real-World Applications of Numerical Sequences

Codes like 74.09 0.23 5-32.32-65 are not just theoretical constructs but are widely used in practical scenarios. In scientific research, for instance, precise measurements are often crucial to testing hypotheses and validating theories. In business, similar codes can help track product prices, supply chain metrics, and market performance indicators.

Additionally, in fields like meteorology, environmental science, and healthcare, numerical sequences are used to represent temperature ranges, patient vitals, or other critical metrics. Their structured format makes it easy to record and compare data across time or locations, helping professionals make informed decisions based on up-to-date information.

The Importance of Consistent Coding Systems

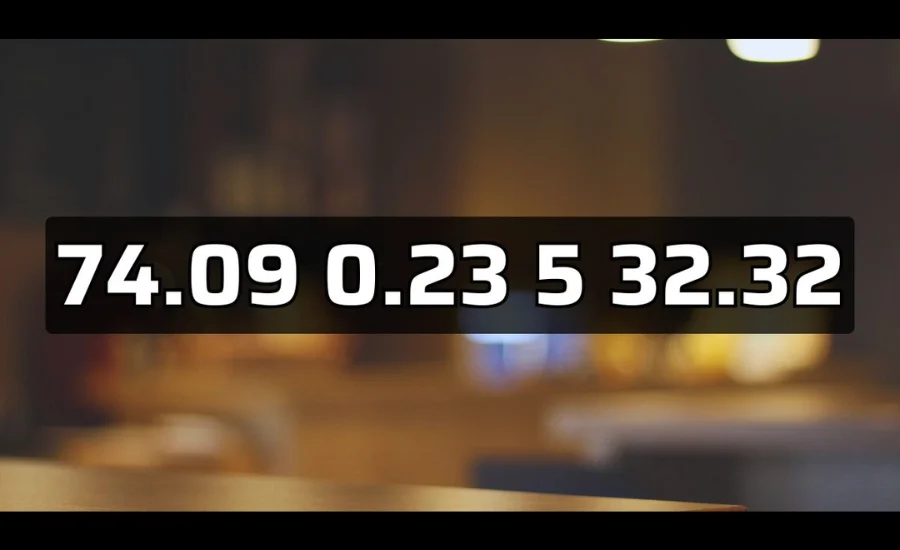
One of the reasons codes like 74.09 0.23 5-32.32-65 are effective is because they follow a consistent structure. This consistency makes it easier for professionals to interpret and work with data over time. Whether you’re reading a temperature gauge, interpreting a financial report, or analyzing scientific results, understanding the structure of numerical codes is essential for ensuring consistency in data collection and reporting.

Also Read: 1-213-720-6488

Final Words

In data analysis, numerical sequences like 74.09 0.23 5-32.32-65 carry more than just raw figures—they unlock valuable insights. These sequences are used across industries, from scientific research to business analytics, to convey essential information efficiently. Each number has a specific role: the first provides a primary measurement, while the following values offer precision, range, or context. This structured approach enhances decision-making, as it allows for quick interpretation of complex data. By recognizing the significance behind these numbers, professionals can identify trends, make predictions, and reduce errors. In an increasingly data-driven world, mastering the interpretation of such sequences is key to making informed, effective decisions.

FAQs

1. What is the significance of numerical sequences in data analysis?

Numerical sequences provide structured and concise information that helps interpret complex data, identify trends, and support decision-making processes across various fields.

2. How do numbers like 74.09, 0.23, and 5-32.32-65 relate to each other?

These numbers represent different aspects of a measurement: 74.09 could be a main data point, 0.23 adds precision, and 5-32.32-65 suggests a range, providing context for variability or fluctuation.

3. Why are decimal numbers used in sequences?

Decimal numbers provide precision, allowing for more accurate measurements or data points, which is essential in scientific research, financial analysis, and other fields that require high accuracy.

4. How does the use of a dash (e.g., 5-32.32) impact data interpretation?

The dash indicates a range, showing that the data point could vary within a specified span. This is particularly useful when data fluctuates or is subject to change.

5. Can numerical sequences be used in financial analysis?

Yes, numerical sequences help track prices, calculate profits, analyze market performance, and assess financial trends, making them essential tools in finance.

6. What role do small numbers, like 0.23, play in data accuracy?

Small values often add fine-tuned detail, correcting or refining measurements. Even a slight change can significantly impact the interpretation of the data.

7. How are numerical sequences applied in scientific research?

In scientific research, these sequences represent measurements, such as temperature, distance, or concentration, and help track variables in experiments or field studies.

8. Why is consistency important in numerical coding?

Consistency in numerical coding ensures that data is interpreted correctly and uniformly across different systems and over time, reducing errors in analysis and reporting.

9. How do ranges in numerical sequences improve decision-making?

Ranges provide flexibility, allowing decision-makers to understand potential variability in the data, which helps manage risks and make adaptable, informed choices.

10. What are the benefits of using numerical sequences in real-time decision-making?

They enable quick, clear, and efficient interpretation of complex data, helping professionals make timely, data-driven decisions in fast-paced environments.

Discover the latest insights and updates tailored for you—only on Insight Graze!